About me
Curriculum vitae
Contact
Welcome to my homepage!
Latest published research (August 2025):
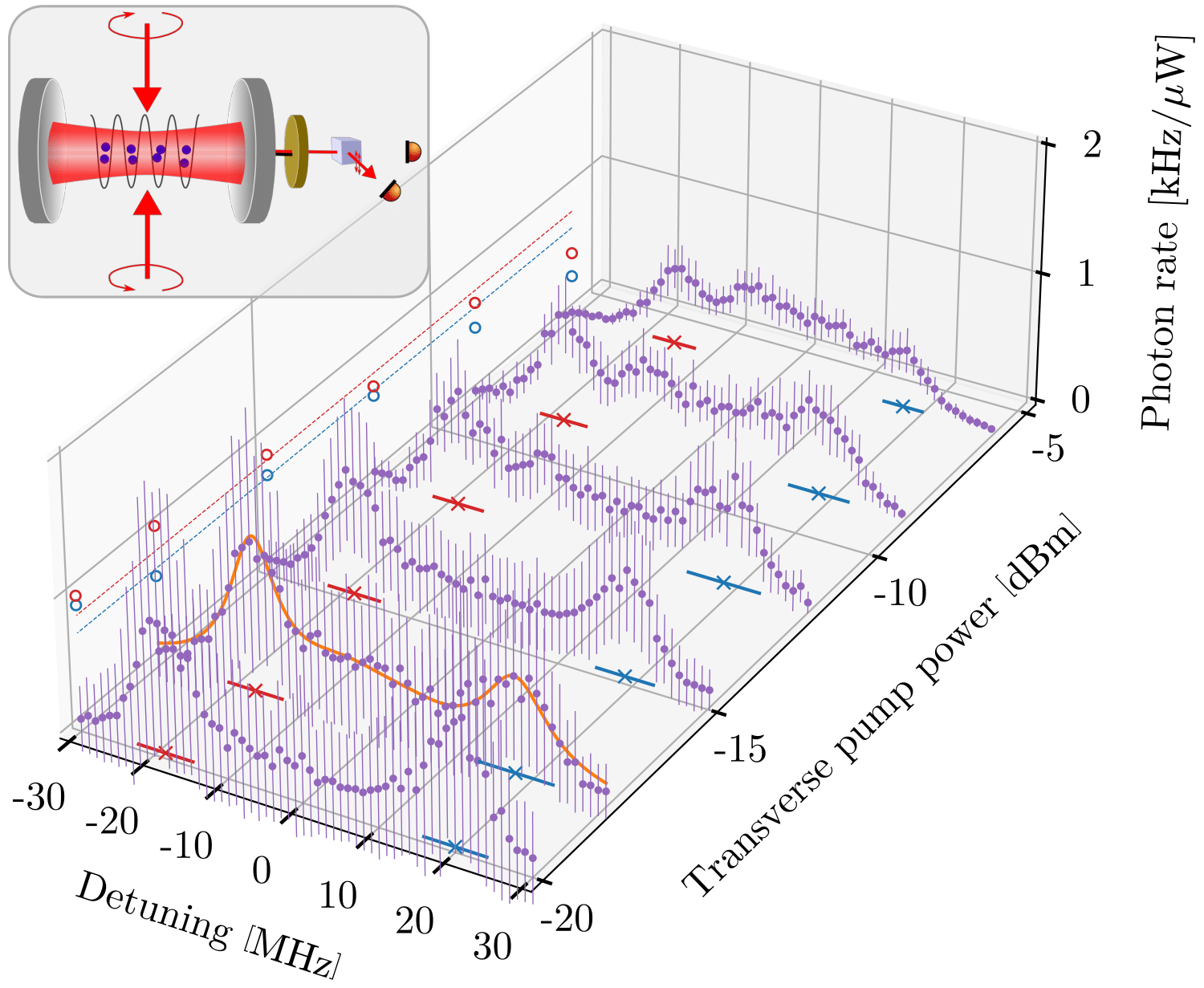
B. Gábor, K. V. Adwaith, D. Varga, B. Sárközi, Á. Kurkó, A. Dombi, T. W. Clark, F. I. B. Williams, D. Nagy, A. Vukics, P. Domokos: Demonstration of strong coupling of a subradiant atom array to a cavity vacuum EPJ Quantum Technol. 12, 93 (2025)
By considering linear (Rayleigh) scattering of cold atoms inside an undriven high-finesse optical resonator, we experimentally demonstrate effects unique to a strongly coupled vacuum field. Arranging the atoms in an incommensurate lattice, with respect to the resonator mode, the scattering can be suppressed by destructive interference: resulting in a subradiant atomic array. We show however, that strong coupling leads to a drastic modification of the excitation spectrum, as evidenced by well-resolved vacuum Rabi splitting in the intensity of the fluctuations. Furthermore, we demonstrate that the strongly coupled vacuum mode induces polarization rotation in the linear scattering, which is incompatible with a linear polarizability model of isotropic objects.